You are here: Concepts>Concepts Web>Class documentation
Class documentation of Concepts
Loading...
Searching...
No Matches
constraints Namespace Reference
Classes | |
| class | AnalyticalConstraint |
| class | ConstraintsList |
| class | Element |
| class | Space |
Detailed Description
Essential boundary conditions and multi-point constraints [1].
The idea is to formulate the constraints as a linear system which has to be fullfilled by the solution:
![\[ Cx = g, \]](form_234.png)
where x is the solution vector, C the constraints matrix and g its right hand side. The assumptions for g and C are as follows:
- Cx = g is solveable, ie. g is in the range of C.
- If a solution exists, it is unique, ie. the intersection of the kernel of C and the kernel of the stiffness matrix is trivial.
- C is of full rank, ie. the conditions are linearly independent.
The last conditions asserts that 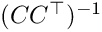
Using C and g, the constraint stiffness matrix is defined by
![\[ S' = C^\top C + Q^\top S Q, \]](form_236.png)
where 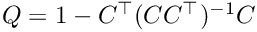
![\[ f' = C^\top g + Q^\top(f - SRg), \]](form_238.png)
where 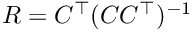
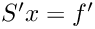
In an application, this could be used in the following way:
constraints::ConstraintsList<Real> constrList;
Add analytical constraints to constrList
Compute C
constraints::Space<Real> constrSpc(spc, constrList);
concepts::SparseMatrix<Real> C(constrSpc, spc, constrList);
concepts::SparseMatrix<Real> Ct(C, true);
Compute CCtinv
concepts::Compose<Real> CtC(Ct, C);
concepts::Compose<Real> CCt(C, Ct);
concepts::SparseMatrix<Real> CCtmatrix(CCt);
concepts::SuperLU CCtinv(CCtmatrix);
Compute R and Q
concepts::Compose<Real> R(Ct, CCtinv);
concepts::Compose<Real> Qmin(R, C);
concepts::LiCoI<Real> Q(Qmin, -1.0);
Compute the stiffness matrix
Identity mass_bf;
concepts::SparseMatrix<Real> mass(spc, mass_bf);
Laplace stiff_bf;
concepts::SparseMatrix<Real> stiff(spc, stiff_bf);
concepts::LiCo<Real> S(stiff, mass);
Compute S'
concepts::Compose<Real> QS(Q, S);
concepts::Compose<Real> QSQ(QS, Q);
concepts::LiCo<Real> Sp(CtC, QSQ);
Compute g and f'
concepts::Vector<Real> g(constrSpc, constrList);
concepts::Vector<Real> Rg(spc);
concepts::Vector<Real> SRg(spc);
R(g, Rg);
S(Rg, SRg);
RHS rhs_lf;
concepts::Vector<Real> f(spc, rhs_lf);
f -= SRg;
concepts::Vector<Real> fp(spc);
Q(f, fp);
Ct(g, SRg);
fp += SRg;
concepts::CG<Real> solver(Sp, 1e-15, 2000);
concepts::Vector<Real> sol(spc);
solver(fp, sol);The following figure gives an overview of the classes in this namespace
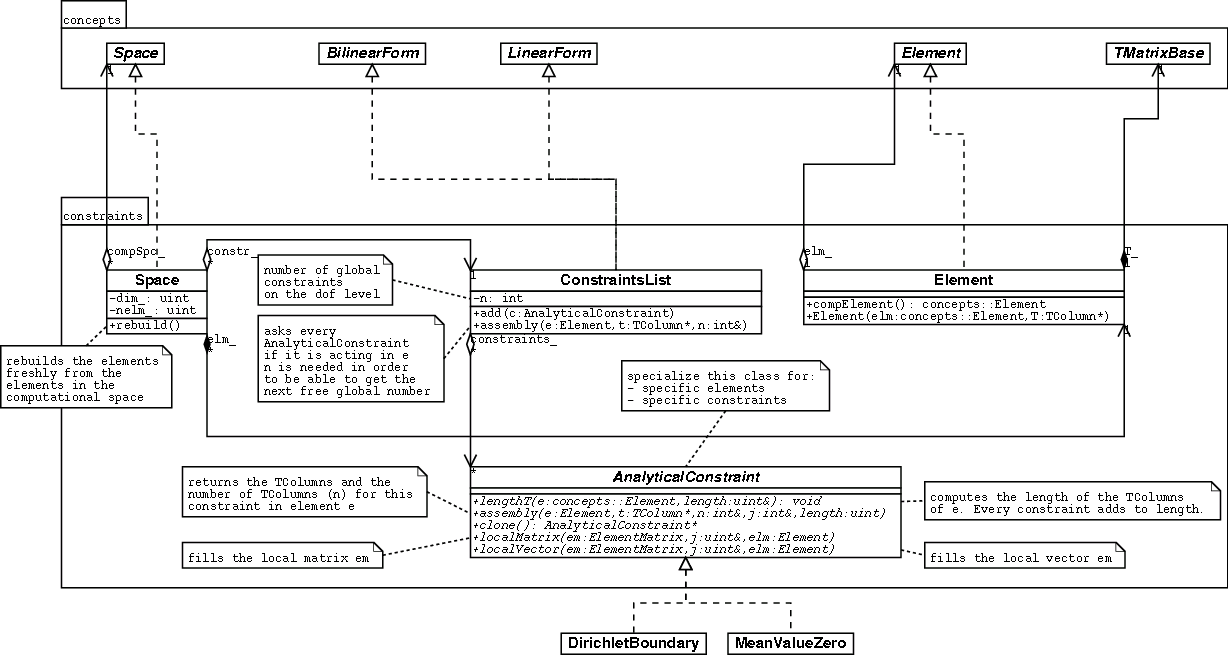
- See also
- Ainsworth, Essential boundary conditions and multi-point constraints in finite element analysis, 2000, Comput. Methods Appl Mech. Engrg. 190 (2001) 6323-6339
Generated on Wed Sep 13 2023 21:06:49 for Concepts by
